Another old assignment here, this one to model the erosion of a theoretical 1cm³ granitoid cube of a given initial composition and neatly present the results. I did the modelling in Python, as one of my earliest Python projects. The plots were made manually in LibreOffice Calc. Below are the plots, for more information, including assumptions and citations, see the short report here. The code used for the model can be found here.
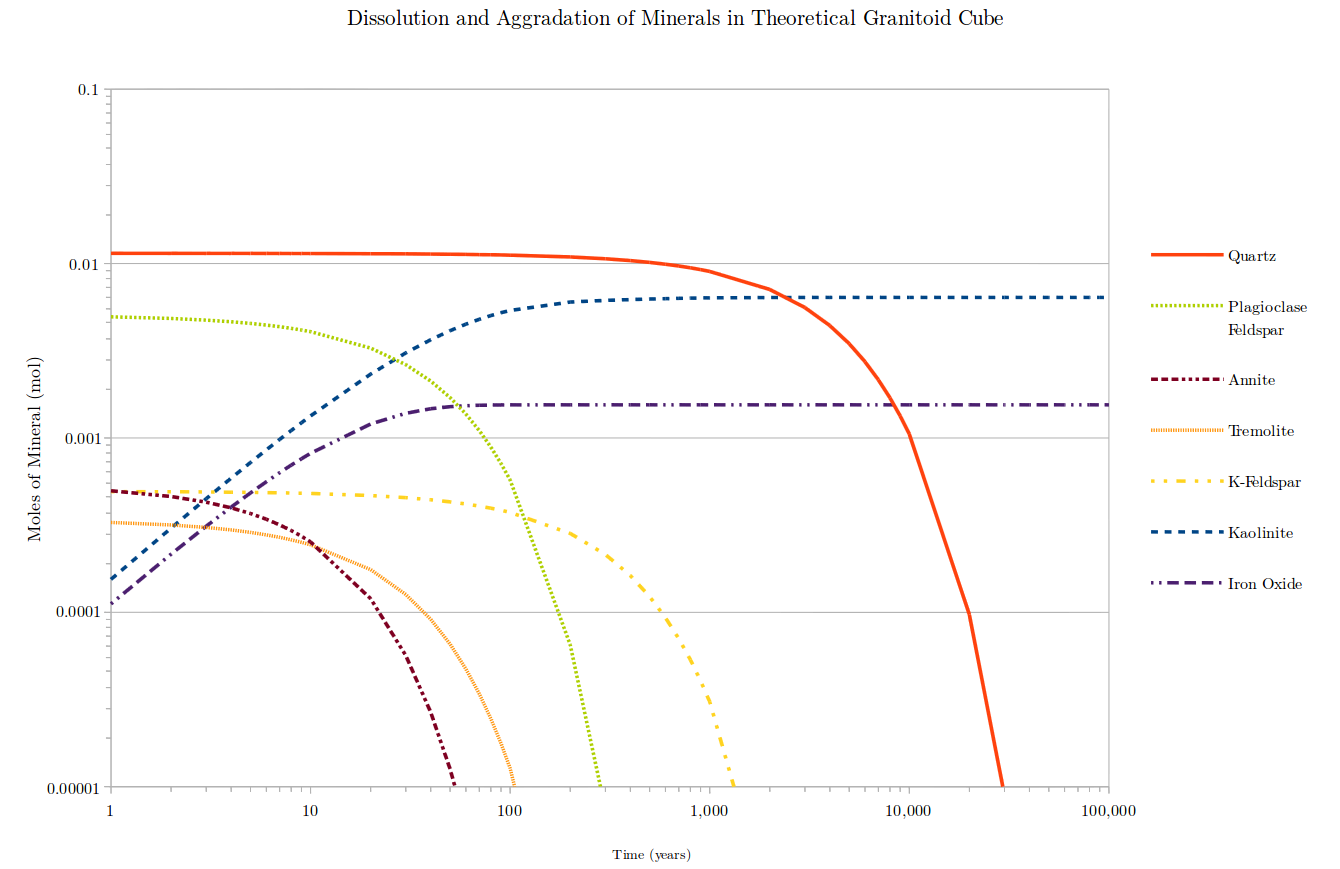
Time dependent log-log plot of dissolution of minerals in a theoretical 1cm 3 granitoid cube in a soil at pH 5, through time. Dissolution rates taken from Bandstra et al. (2007) and Palandri and Kharaka (2004). Initial weathering rates of all minerals at the start of the experiment (quartz, plagioclase feldspar, annite, tremolite, and potassium feldspar) are faster than all subsequent weathering rates rates due to continually decreasing surface area of the mineral through time. Aggradation of kaolinite is dependent on the dissolution rates of plagioclase feldspar, potassium feldspar and annite. Iron oxide aggradation is dependent on annite weathering rate. Minerals reach "zero value point" at 0.00001 moles of mineral. These times are roughly 50 years for annite, 100 years for tremolite, 300 years for plagioclase feldspar, 1,500 years for potassium feldspar, and 30,000 years for quartz.
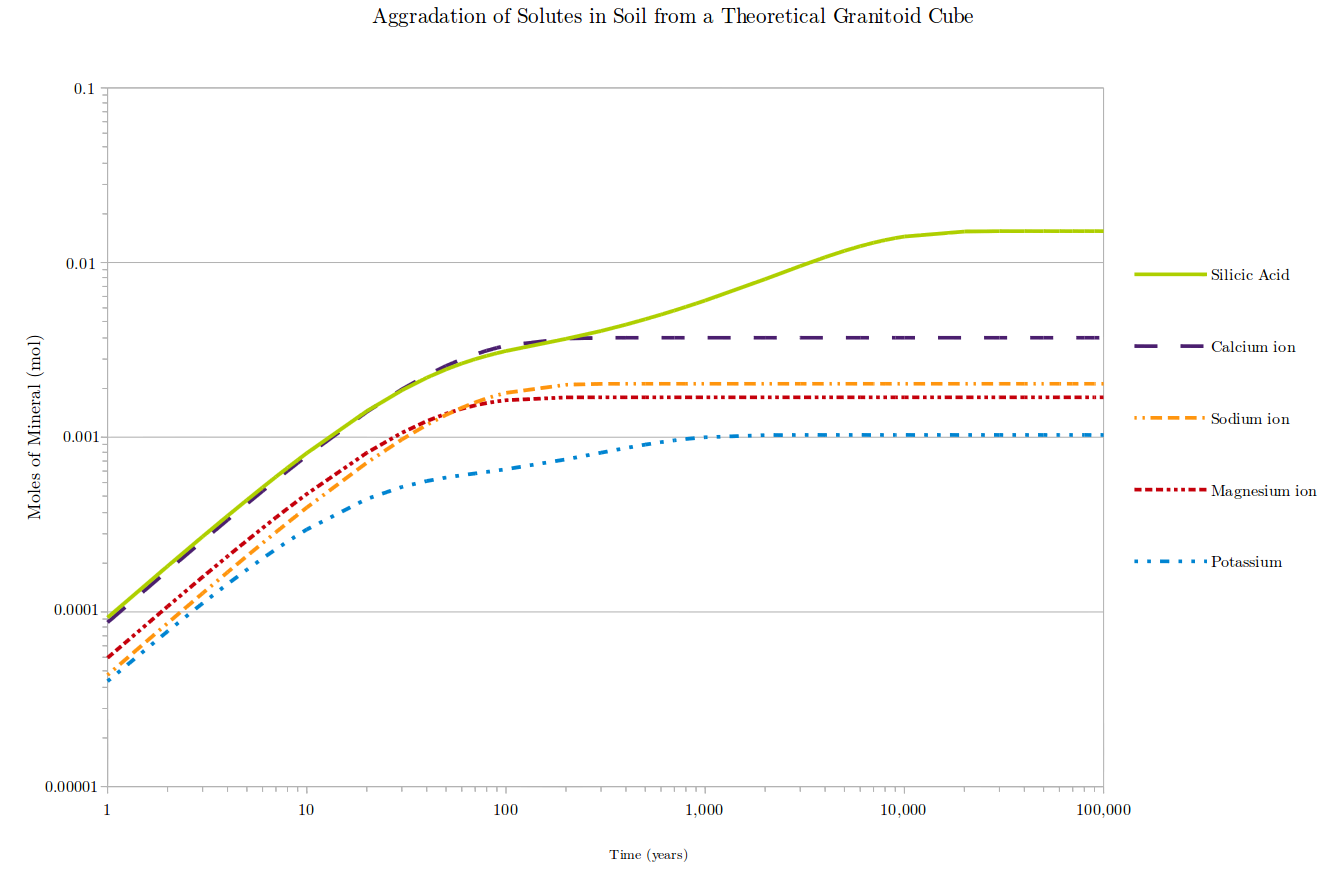
The aggradation of solutes from the same granitoid cube described in Figure 1, in a soil. The aggradation is entirely dependent on the weathering rates of the primary minerals in the granitoid cube. Plagioclase feldspar yields calcium and sodium ions into solution, quartz yields silicic acid, annite yields potassium ions, tremolite yields calcium and sodium ions and silicic acid, and potassium feldspar yields potassium ions and silicic acid.


